The Delboeuf, Ponzo and More Illusions
By Grant Ocean
Other Size Illusions
Before we end this article, let's use the principle: The
smaller portion of the visual field an object occupies, the smaller the object appears to be, and vice versa, to explain two more illusions. The first one is called the Occlusion
illusion, in which a figure bounded by an occluding edge looks larger
than the same figure not bounded by an occluder, as shown in the figure
 on
the left below. The most acceptable explanation, like all the other
size illusion figures, is that it is caused by perspective. The gray
bar seems to be in front of a solid black circle; hence the semicircle
we can see is behind the gray bar and it must be farther away from the
viewer in comparison to the semicircle which is not blocked by
anything. According to the perspective hypothesis
the faraway object looks larger than a nearer object of the same
retinal size. The distance and perspective are the factors that we have
already dismissed as contributing to the size illusions with reasons
listed above. If the above principle is correct, all we need to do is
to expand the visual field (or the sub-visual field to be specific) to
decrease the portion of the semicircle in the visual field in order to
make it appear smaller. I think that I have achieved the
expected result by lengthening the gray bar to make the semicircle
appear smaller, as shown in the figure on the right below. Lengthening
the gray vertical bar for the occlusion illusion is the equivalent of
widening the road for the lower red bar for the famous Ponzo illusion
picture on the right. As a result, the sub-visual field around the
objects has been expanded. At this point, someone might ask why the
semicircle that is not occluded by anything looks small; in fact it
looks even smaller than the semicircle occluded by the lengthened bar.
I think that it looks smaller because our brain treats the whole page,
from the top to the bottom, on the left side of the semicircle is
blocking it. Thus, we have a much larger sub-visual field for the
unbounded semicircle so that it appears to be much smaller according to
the principle. As a matter of fact, this understanding of the unbounded semicircle
gave me the idea of lengthening the vertical gray bar to expand the
visual field in the first place.
on
the left below. The most acceptable explanation, like all the other
size illusion figures, is that it is caused by perspective. The gray
bar seems to be in front of a solid black circle; hence the semicircle
we can see is behind the gray bar and it must be farther away from the
viewer in comparison to the semicircle which is not blocked by
anything. According to the perspective hypothesis
the faraway object looks larger than a nearer object of the same
retinal size. The distance and perspective are the factors that we have
already dismissed as contributing to the size illusions with reasons
listed above. If the above principle is correct, all we need to do is
to expand the visual field (or the sub-visual field to be specific) to
decrease the portion of the semicircle in the visual field in order to
make it appear smaller. I think that I have achieved the
expected result by lengthening the gray bar to make the semicircle
appear smaller, as shown in the figure on the right below. Lengthening
the gray vertical bar for the occlusion illusion is the equivalent of
widening the road for the lower red bar for the famous Ponzo illusion
picture on the right. As a result, the sub-visual field around the
objects has been expanded. At this point, someone might ask why the
semicircle that is not occluded by anything looks small; in fact it
looks even smaller than the semicircle occluded by the lengthened bar.
I think that it looks smaller because our brain treats the whole page,
from the top to the bottom, on the left side of the semicircle is
blocking it. Thus, we have a much larger sub-visual field for the
unbounded semicircle so that it appears to be much smaller according to
the principle. As a matter of fact, this understanding of the unbounded semicircle
gave me the idea of lengthening the vertical gray bar to expand the
visual field in the first place.


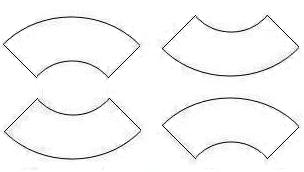
The next and the last size illusion we are going to take
a look at is the Jastrow illusion. In this size illusion as shown on
the right, the lower figure looks slightly bigger than the figure above
even though they are exactly the same size. This is a poorly understood phenomenon. Thus far
nobody could explain it with reasonable success. The researchers could
not even relate this illusion to perspective. All they could say is that
the illusion is due to the fact that the longer upper side of the lower
figure is somehow compared with the shorter lower side of the upper
figure, which makes the lower figure look larger as the result
of this comparison. As far as the actual mechanism of this comparison
is concerned, nobody knows. By looking at the diagram below, we all
would agree that a longer side of one figure facing a shorter side of
another is a contributing factor in the illusion because the illusory
effect disappears when we turn one figure around so that either the
shorter sides of both figures or the longer sides of both figures are
facing each other. This is a fact everybody knows; but the reason why it is perceived as such is
a mystery.
exactly the same size. This is a poorly understood phenomenon. Thus far
nobody could explain it with reasonable success. The researchers could
not even relate this illusion to perspective. All they could say is that
the illusion is due to the fact that the longer upper side of the lower
figure is somehow compared with the shorter lower side of the upper
figure, which makes the lower figure look larger as the result
of this comparison. As far as the actual mechanism of this comparison
is concerned, nobody knows. By looking at the diagram below, we all
would agree that a longer side of one figure facing a shorter side of
another is a contributing factor in the illusion because the illusory
effect disappears when we turn one figure around so that either the
shorter sides of both figures or the longer sides of both figures are
facing each other. This is a fact everybody knows; but the reason why it is perceived as such is
a mystery.
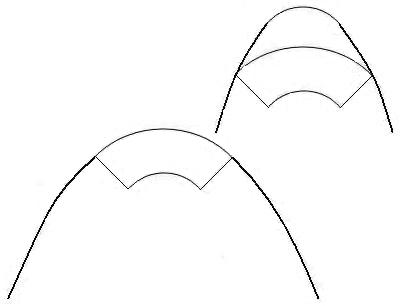
As I said earlier, I am going to use the principle: The
smaller portion of the visual field an object occupies, the smaller the object appears to be, and vice versa, to
explain the illusion. The upper figure and the lower figure must be
enclosed in a differently sized sub-visual fields. As shown in the
diagram on the left below, both the upper figure and the lower figure
are enclosed in the extended curve lines of the upper figure's curved
sides. I also tried to extend the curved sides of the lower figure; but
the extended curve lines of the lower figure do not have any illusory
effect on the figures. Therefore, I have only kept the extended curve
lines from the upper figure. Now let's look at the diagram on the right
below. I have separated the two figures with their surrounding extended
lines. Thus, the figures are inside their separate sub-visual fields. I
have placed the lower figure on top and the upper figure at bottom in
the diagram on the right below, in an attempt to eliminate the effect of
a longer side facing a shorter side as in the original illusion.
As predicted by the principle, the top figure looks bigger because it
is in a smaller visual field than the bottom figure. Some readers might
not buy my explanation of the Jastrow illusion and might think that it
is far-fetched. The only way I can convince them is to urge them to
take a look at the magnitudes of the size difference between the two
figures in the diagram on the right below and compare with the
magnitudes of the size difference in the original illusion figure. The
explanation should be deemed credible if there is a close match between
them. It is up to you to decide.

I have used the principle that the
smaller portion of the visual field an object occupies,
the smaller the object appears to be, and vice versa to explain some of
the size illusions which are depicted usually by the 2-D drawings or
pictures. You can also test this principle in the real world. Join your
two hands with curved fingers to make a large tube; then view an object through this hand tube with one eye and the other eye closed.
Pay close attention to the edges of the hand tube while viewing the
object, and you will find that the object appears smaller than before.
Now make a smaller tube by cupping one hand and view the same object through this cupped hand.
You will find that the object appears to become larger than when viewed
through the larger hand tube. Thus, the sub-visual field volumes
created by our own hands in the real world can make the real objects
appear larger or smaller following the above principle.
I
have discussed some of the most important size illusions in this
article, and we have gained a quite different outlook from the
conventional wisdom. Some of the issues are far-reaching and could be
even fundamental to our understanding of the perception, our mind, and
perhaps the world. Also, some of these issues are very complicated,
with which you probably feel that I haven't dealt thoroughly. You are
right that it is impossible for an article of this length to discuss
all these important issues adequately. Therefore, I am going to further
discuss these issues in the next articles. Finally, all the discussions
will lead us to the most important size illusion, the Moon illusion.
This article is just the beginning. We have a long way to go!
TOP
Back to the Delboeuf Illusion Index
References
Rock, I. and Ebenholtz, S. (1959). The relational determination of
perceived size. Psychol. Rev., 66, 387-401
Broerse J. et al. (1992). The apparent shape of afterimages in the Ames room. Perception, 21(2): 261-8.
Related Information on the Web:
http://www.newworldencyclopedia.org/entry/Ponzo_illusion
http://www.gla.ac.uk/sums/users/Maxine/Ponzo/instruct2.html
http://en.wikipedia.org/wiki/Ebbinghaus_illusion
 on
the left below. The most acceptable explanation, like all the other
size illusion figures, is that it is caused by perspective. The gray
bar seems to be in front of a solid black circle; hence the semicircle
we can see is behind the gray bar and it must be farther away from the
viewer in comparison to the semicircle which is not blocked by
anything. According to the perspective hypothesis
the faraway object looks larger than a nearer object of the same
retinal size. The distance and perspective are the factors that we have
already dismissed as contributing to the size illusions with reasons
listed above. If the above principle is correct, all we need to do is
to expand the visual field (or the sub-visual field to be specific) to
decrease the portion of the semicircle in the visual field in order to
make it appear smaller. I think that I have achieved the
expected result by lengthening the gray bar to make the semicircle
appear smaller, as shown in the figure on the right below. Lengthening
the gray vertical bar for the occlusion illusion is the equivalent of
widening the road for the lower red bar for the famous Ponzo illusion
picture on the right. As a result, the sub-visual field around the
objects has been expanded. At this point, someone might ask why the
semicircle that is not occluded by anything looks small; in fact it
looks even smaller than the semicircle occluded by the lengthened bar.
I think that it looks smaller because our brain treats the whole page,
from the top to the bottom, on the left side of the semicircle is
blocking it. Thus, we have a much larger sub-visual field for the
unbounded semicircle so that it appears to be much smaller according to
the principle. As a matter of fact, this understanding of the unbounded semicircle
gave me the idea of lengthening the vertical gray bar to expand the
visual field in the first place.
on
the left below. The most acceptable explanation, like all the other
size illusion figures, is that it is caused by perspective. The gray
bar seems to be in front of a solid black circle; hence the semicircle
we can see is behind the gray bar and it must be farther away from the
viewer in comparison to the semicircle which is not blocked by
anything. According to the perspective hypothesis
the faraway object looks larger than a nearer object of the same
retinal size. The distance and perspective are the factors that we have
already dismissed as contributing to the size illusions with reasons
listed above. If the above principle is correct, all we need to do is
to expand the visual field (or the sub-visual field to be specific) to
decrease the portion of the semicircle in the visual field in order to
make it appear smaller. I think that I have achieved the
expected result by lengthening the gray bar to make the semicircle
appear smaller, as shown in the figure on the right below. Lengthening
the gray vertical bar for the occlusion illusion is the equivalent of
widening the road for the lower red bar for the famous Ponzo illusion
picture on the right. As a result, the sub-visual field around the
objects has been expanded. At this point, someone might ask why the
semicircle that is not occluded by anything looks small; in fact it
looks even smaller than the semicircle occluded by the lengthened bar.
I think that it looks smaller because our brain treats the whole page,
from the top to the bottom, on the left side of the semicircle is
blocking it. Thus, we have a much larger sub-visual field for the
unbounded semicircle so that it appears to be much smaller according to
the principle. As a matter of fact, this understanding of the unbounded semicircle
gave me the idea of lengthening the vertical gray bar to expand the
visual field in the first place. 




